Eine Zug-Analogie: Strömungs- und Druckwellen in Fluidsystemen

Druck- oder Durchflusspulsationen können bestimmte Anwendungen negativ beeinflussen. Beim Inkjetdruck beispielsweise verringern bereits moderate Druckpulsationen den Wirkungsgrad und die maximale Durchflusskapazität des Tintenzufuhrsystems. In Systemen, in denen die Druckpulsationen einen höheren Druck erreichen, wächst das Risiko für gravierendere Probleme wie Schaumbildung und Tintenmangel, was die Druckqualität beeinträchtigt. Hohe Druckpulsationen haben zudem einen negativen Einfluss auf die Lebensdauer und Zuverlässigkeit der Komponenten.

KNF hat eine neue FP-Pumpenserie mit geringer Pulsation entwickelt, die selbst bei anspruchsvolleren Anwendungen eine weitaus gleichmäßigere Förderleistung erreicht und die oben genannten Probleme beseitigt. Der Entwicklungsprozess dieser FP-Pumpen wurde durch den Einsatz eines neuen firmeneigenen Simulationstools erheblich beschleunigt. Mithilfe dieses Tools lassen sich Durchfluss- und Druckabweichungen in Fluidsystemen präzise vorhersagen.

Die Rolle des Schlauchs innerhalb eines Fluidsystems

Das Schlauchverhalten zu kennen ist entscheidend, um zu bestimmen, wie sich eine pulsierende Strömung – wie sie zum Beispiel von einer einköpfigen Membranpumpe verursacht werden kann – auf ein Fluidsystem auswirkt. Dieser Artikel zeigt, welche physikalischen Effekte das Verhalten von Schläuchen beeinflussen, die pulsierende Strömungen führen. Es handelt sich dabei um die folgenden Effekte:

• Flüssigkeitsreibung (Widerstand)
• Trägheit der Flüssigkeitssäule innerhalb des Schlauchs
• Kapazität der Schlauchwand

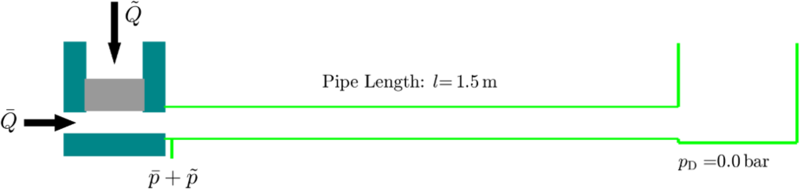
Mithilfe eines virtuellen Experiments wird die dem jeweiligen Effekt zugrunde liegende Physik veranschaulicht und verdeutlicht. Das Experiment (siehe Abbildung 1), umfasst einen Schlauch aus Weich-Polyvinylchlorid (PVC-P) mit einer Länge \(l\) von 1,5 m, einem Innendurchmesser \(d\) von 4 mm und einer Wanddicke von 1 mm. Das nachgeschaltete Ende des Schlauchs ist mit einem Reservoir verbunden, das Wasser unter Umgebungsluftdruck enthält. Am vorgeschalteten Ende des Schlauchs ist ein Kolben angebracht, der eine pulsierende Strömung \(\tilde{Q}\) erzeugt und in den Schlauch einleitet. Zudem wird über einen separaten Einlass \(\overline{Q}\) eine gleichmäßige Strömung an den Schlauch abgegeben. Zwei Animationen zu diesem Experiment sind in Video 1 und 2 zu sehen.

Wir werden die Reaktion des Schlauchs auf die Kombination aus gleichmäßigen und ungleichmäßigen Strömungen am vorgeschalteten Ende des Schlauchs an der mit einem grünen Strich markierten Stelle beobachten. An dieser Stelle wird die Summe aus gleichmäßigem \((\overline{p})\) und ungleichmäßigem \((\tilde{p})\) Druckbeitrag gemessen. Das Verhältnis zwischen dem an dieser Stelle gemessenen Druck und der eingeleiteten Strömung ist die hydraulische Impedanz \(Z\) des Schlauchs, die mit der Hubfrequenz \(n\) des Kolbens variiert.

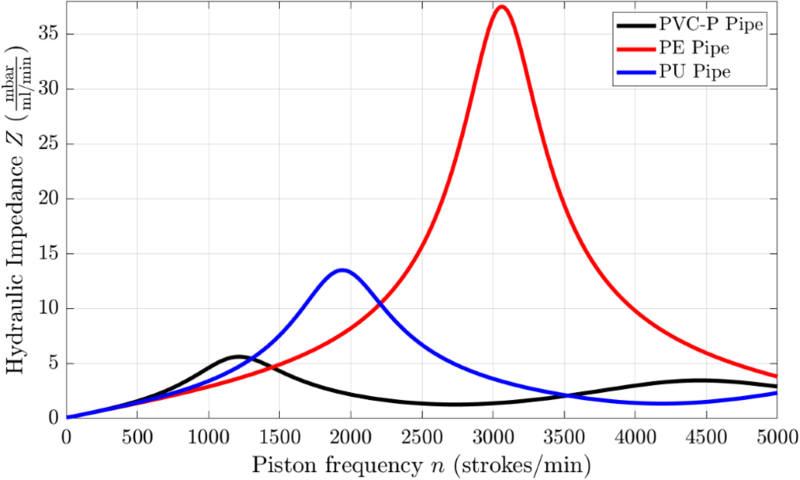
Der gesamte Impedanzverlauf des Schlauchs bei Frequenzen von bis zu 5.000 Hüben pro Minute wird in Abbildung 2 als durchgezogene schwarze Kurve dargestellt. Dieser Impedanzverlauf berücksichtigt alle drei der oben genannten Effekte (Widerstand, Induktivität, Kapazität) und repräsentiert das tatsächliche Verhalten des Schlauchs. Im Folgenden wird jeder physikalische Effekt einzeln untersucht.

Flüssigkeitsreibung

Als Erstes wird die Flüssigkeitsreibung bzw. der Schlauchwiderstand untersucht. Dieser Effekt wird durch Reibungseffekte zwischen der Schlauchwand und der durch den Schlauch strömenden Flüssigkeit verursacht. Für diesen Effekt werden normalerweise zwei Formen des Druckbeitrags betrachtet: ein stationärer (kontinuierlicher) und ein instationärer (pulsierender) Druckbeitrag. Das physikalische Gesetz, das diesen stationären Druckbeitrag beschreibt, ist allgemein bekannt und besagt, dass sich der Druckverlust in Schläuchen bei einer kontinuierlich strömenden Flüssigkeit proportional zur Strömung im Schlauch verhält (Hagen, 1839; Poiseuille, 1841), vorausgesetzt, der Strömungszustand ist laminar.

\(\begin{equation}\nonumber \bar{p} = \frac{128\eta l}{d^4\pi}\bar{Q} \end{equation}\)

Wobei \(\eta\) die dynamische Viskosität der Flüssigkeit im Schlauch beschreibt. Somit ist der Widerstand bei stationärem Druckbeitrag konstant und unabhängig von der Kolbenfrequenz (siehe Abbildung 2, gestrichelte rote Linie). Zudem lässt sich beobachten, dass dieser Druckbeitrag angesichts der tatsächlich gemessenen Impedanz (schwarze Kurve) verhältnismäßig klein ist. Nur bei Frequenz Null ist der tatsächliche Impedanzverlauf (schwarze Kurve) identisch mit dem gleichförmigen Widerstand des Schlauchs (gestrichelte rote Linie). Die Kombination aus stationärer und instationärer Reibung wird in Abbildung 2 als durchgezogene rote Linie dargestellt. Der instationäre Druckbeitrag ist weniger bekannt. Es handelt sich um ein frequenzabhängiges, nicht-lineares Gesetz, das Druckverlust und Strömung im Schlauch in Beziehung zueinander setzt (Zielke, 1968). Wie jedoch aus Abbildung 2 ersichtlich wird, ist der kombinierte Widerstandseffekt bei den meisten Kolbenfrequenzen weiterhin klein, verglichen mit der tatsächlich gemessenen Impedanz des PVC-P-Schlauchs.

Ein schnell fahrender Zug auf gerader Strecke ist eine gute Analogie, um diese Effekte rund um den Flüssigkeitswiderstand zu veranschaulichen. Stellen Sie sich zunächst vor, dass ein Zug mit konstanter Geschwindigkeit fährt. Die den Zug umgebende Luft verursacht Reibung – eine konstante Kraft, die es für den Motor des Zuges zu überwinden gilt. Dann stellen Sie sich vor, dass der Zugführer den Zug in harmonischer Weise beschleunigt und abbremst. Dadurch entsteht ein zusätzlicher instationärer Reibungseffekt.

Trägheit der Flüssigkeitssäule

In diesem Abschnitt werden lediglich Effekte mit Bezug auf die Masse der Flüssigkeitssäule innerhalb des Schlauchs betrachtet. Massebezogene Effekte werden als Trägheitseffekte bezeichnet. Mithilfe der oben verwendeten Zug-Analogie kann dieser Effekt relativ einfach veranschaulicht werden. Angenommen der Zug bewegt sich in einem gleichmäßigen Schwingungsmuster auf einer geraden Zugstrecke vor und zurück. Bewegt sich der Zug langsam, ist das durch den Motor aufzuwendende Drehmoment gering. Bei zunehmender Schwinggeschwindigkeit des Zuges ist allerdings ein immer höheres Drehmoment erforderlich.

Dies gilt auch für den Druckaufbau im unter Abbildung 1 gezeigten Experiment. Bei niedriger Schwingfrequenz des Kolbens ist der Druckaufbau im Schlauch gering. Sobald die Schwingfrequenz ansteigt, steigt jedoch der Druck proportional zur angelegten Frequenz. Die daraus resultierende Impedanz in einem solchen Fall wird als durchgezogene blaue Linie in Abbildung 2 dargestellt. Überraschenderweise verursacht der Trägheitseffekt eine deutlich höhere Impedanz (bzw. Druckpulsationen) als der Widerstandseffekt. Zusätzlich lässt sich beobachten, dass sich bei niedrigen Kolbenfrequenzen von bis zu 500 Hüben pro Minute der tatsächliche Impedanzverlauf und die Kurve, die den Trägheitseffekt im Schlauch darstellt, beinah decken. Bei höheren Kolbenfrequenzen werden die Unterschiede allerdings zunehmend größer und der blaue lineare Impedanzverlauf gibt nicht die tatsächliche nicht-lineare Impedanz des PVC-P-Schlauchs in Form der schwarzen Kurve wieder. Es muss also einen zusätzlichen Effekt geben, der dieses besondere Verhalten von Polymer-Schläuchen hervorruft.

Rohrwandkapazität

Das charakteristische, nicht-lineare Verhalten von Schläuchen, dargestellt durch die schwarze Kurve in Abbildung 2, lässt sich nur unter Berücksichtigung der Elastizität der Schlauchwand erklären. Wichtig ist hierbei die Tatsache, dass sich die Schlauchwand geringfügig ausdehnt und zusammenzieht und somit eine bestimmte Flüssigkeitsmenge entweder durch die Schlauchwand aufgenommen oder abgegeben wird, abhängig vom vorherrschenden Druck an dieser Stelle des Schlauchs. Aus diesem Grund wird dieser Effekt als Kapazitätseffekt bezeichnet. Man kann sich vorstellen, dass der Schlauch eine Reihe kleiner Kondensatoren enthält, die gleichmäßig entlang des Schlauchs verteilt sind.

Auch hier kann wieder die Zug-Analogie angewendet werden, um diesen Kapazitätseffekt zu erklären, der in starker Wechselwirkung zum im vorherigen Abschnitt beschriebenen Trägheitseffekt steht. Stellen Sie sich erneut den Zug bestehend aus einem Triebwagen und mehreren Waggons auf gerader Strecke vor. Dieses Mal wird allerdings anstelle einer starren Kupplung zwischen den Waggons eine elastische Verbindung wie ein Feder- oder Gummiband verwendet. Jetzt bewegt sich der Triebwagen mit einer Schwingbewegung vor und zurück. Bei niedrigen Frequenzen bewegen sich die Waggons synchron (in Phase) mit dem Triebwagen und das Verhalten des Zuges gleicht dem Fall mit einer starren Kupplung zwischen den Waggons. Erhöht sich die Schwingungsfrequenz des Triebwagens, treten die Waggons und Kupplungen miteinander in Wechselwirkung und die Bewegungen des Triebwagens und der Waggons werden asynchron. Ein solcher Fall wird in Video 1 dargestellt. Es handelt sich um dasselbe Experiment, das in Abbildung 1 vorgestellt wurde, allerdings mit einer Kolbenfrequenz von 1.200 Hüben/min.

Die grünen Sterne markieren den schwingenden Teil der Bewegung flüssiger Partikel am Anfang, in der Mitte und am Ende des Schlauchs. Wenn Sie sich den ersten Stern (den Triebwagen) und den letzten Stern (den letzten Waggon des Zuges) ansehen, lässt sich beobachten, dass die Bewegung der Partikel an diesen Stellen asynchron (phasenverschoben) ist. In diesem speziellen Fall beträgt die Phasendifferenz zwischen dem ersten und dem letzten Flüssigkeitspartikel 90°. Weiterhin wird die Druckverteilung entlang der Schlauchleitung als Ausdehnung der Schlauchwand visualisiert. Bei der gewählten Kolbenfrequenz in Video 1 lässt sich beobachten, dass die Bewegung des Schlauchs gegen den Kolben läuft und somit der Impedanzwert bei 1.200 Hüben/min und die entsprechenden Druckpulsationen, wie durch die grüne Kurve in Video 1 dargestellt, sehr hoch und weitaus größer sind als der reine aus Widerstand und Trägheit kombinierte Effekt.

Wird die Kolbenfrequenz weiter erhöht, ist es möglich, dass wenn sich der Triebwagen zurück- und vorbewegt, der letzte Waggon des Zuges vor- und zurückbewegt (180°, phasenverschoben). Ein solcher Fall wird in Video 2 gezeigt, in dem die Kolbenfrequenz 2.700 Hüben/min beträgt. Mit Blick auf den ersten Stern (Triebwagen) und den letzten Stern (letzter Waggon des Triebwagens) lässt sich beobachten, dass die Partikelbewegung an diesen Stellen um exakt 180° phasenverschoben ist. Erneut wird die Druckverteilung entlang der Schlauchleitung als Ausdehnung der Schlauchwand visualisiert. In diesem besonderen Fall scheint der Schlauch mit dem Kolben zu wirken, weshalb der Impedanzwert bei 2.700 Hüben/min liegt und die Druckpulsation am Schlaucheingang in Form der grünen Kurve erheblich geringer ausfällt (im Vergleich zum Ergebnis bei 1.200 Hüben/min).

Diese zwei besonderen Fälle zeigen deutlich, wie im Schlauch Druck- und Strömungswellen entstehen und mit dem Kolben in Wechselwirkung treten. Diese Wellenphänomene sind der Grund für die besondere Form der Impedanzkurve, wie in Abbildung 2 gezeigt. Die Form der Impedanzkurve hängt stark von den Materialeigenschaften der Schläuche ab, wie aus Abbildung 3 für die Schlauchmaterialien Polyethylen (PE), Polyurethan (PU) und PVC-P ersichtlich wird. Diese Materialeigenschaften können durch eine spezielle Messmethode ermittelt werden, die bei KNF (Frey et al., 2020) entwickelt wurde.

Übertragung auf eine reale Pumpensimulation

Obwohl das oben gezeigte Verhalten von Polymer-Schläuchen schwierig zu erfassen ist, ist es nur ein Schritt in Richtung einer vollständigen Fluidsystem-Simulation inklusive Membranpumpen. Für eine vollständige Systembeschreibung müssen zusätzliche Wechselwirkungen zwischen dem vorgeschalteten Schlauch und der Pumpe, dem nachgeschalteten Schlauch und der Pumpe sowie das gesamte Pumpenverhalten berücksichtigt werden. Ein solches Simulationstool wurde bei KNF entwickelt und wird für die Konstruktion neuer Pumpen verwendet, wie in einem der folgenden Posts auf dem KNF-Blog gezeigt werden wird. Weiterhin kann dieses Simulationstool verwendet werden, um KNF-Pumpen in Kundensystemen auszuwerten, sofern detaillierte Systeminformationen vorliegen. Die Kenntnis der hydraulischen Impedanz von Schläuchen zeigt allerdings bereits das große Potenzial, welches sich für Pumpen mit einem gleichmäßigen Förderstrom ergibt. Bei einem kontinuierlichen Durchfluss mit einer Strömungspulsation von Null ist die Schlauchimpedanz minimal (siehe die gepunktete rote Linie in Abbildung 2) und identisch mit dem Schlauchwiderstand, der sich einfach berechnen lässt. Bei ungleichmäßigem Durchfluss ändert sich die Impedanz des Schlauchs mit der Frequenz. Die Abweichung kann recht hoch sein, wenn zur Ermittlung der Systemimpedanz lediglich der Schlauchwiderstand berücksichtigt wird, wie dies zum Beispiel bei der schwarzen Kurve in Abbildung 2 bei einer Frequenz von 1.200 Hüben/min der Fall ist.

Wir hoffen, dass dieser technische Blog hilft, zu verstehen, warum in manchen Fällen Wellenphänomene große Druck- oder Durchflusspulsationen in Fluidsystemen verursachen können. Die Experten bei KNF verfügen über umfassendes Wissen zu diesen Fällen. Sollten Sie auf ein Problem stoßen, zögern Sie nicht, sich an das zuständige Vertriebszentrum zu wenden. Wir werden versuchen, eine Lösung für Ihren Fall zu finden.

Literatur

Frey, R., Güdel, M., Dual, J., & Staubli, T. (2020). Phase and amplitude based characterization of small viscoelastic pipes in the frequency domain with a reservoir–pipeline–oscillating-valve system [Phasen- und amplitudenbasierte Charakterisierung von kleinen viskoelastischen Schläuchen im Frequenzbereich mit einem Reservoir-Schlauch-Schwingungs-Ventil-System]. Journal of Hydraulic Research, 58(3), 460-470.

Hagen, G. (1839). Über die Bewegung des Wassers in engen zylindrischen Röhren. Annalen der Physik (46), 432–442.

Poiseuille, J. L. (1841). Recherches expérimentales sur le mouvement des liquides dans les tubes de très-petits diamètres [Experimentelle Untersuchungen zur Bewegung von Flüssigkeiten in Schläuchen mit sehr kleinen Durchmessern]. Comptes Rendus de l’Académie des sciences.

Zielke, W. (1968). Frequency-dependent friction in transient pipe flow [Frequenzabhängige Reibung in instationärer Schlauchströmung]. Journal of Basic Engineering, 90 (1), 109–115.